Calculations of Strings Downforce on the Bridge:
$T\small(kg_{F})\normalsize = T\small(lb_{F})\normalsize \times 0.45359$
$\cos\alpha + \cos\beta = 2\cos(\frac{\alpha + \beta}{2})\cos(\frac{\alpha - \beta}{2})$
$F_{d}\small(kg_{F})\normalsize = T\small(kg_{F})\normalsize \times 2\cos(\frac{\alpha + \beta}{2})\cos(\frac{\alpha - \beta}{2})$
$F_{d}\small(N)\normalsize = F_{d}\small(kg_{F})\normalsize \times 9.80665$
$T$ = String Tension
$F_{d}$ = Downforce on the Bridge
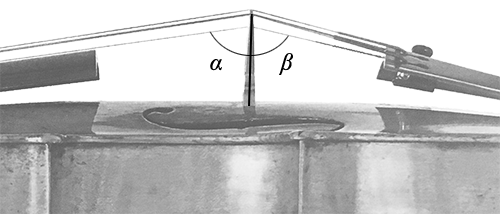
Among the many equations that are used to determine the downforce applied by the strings to the bridge, foresaid equation encompasses a more general condition in which the angle of the string on two sides of the bridge can take different values. In the picture, the direction of the calculated force (corresponding to the central axis of the bridge) is shown. If a different direction is desired for the calculated force, use of vector calculation is required. It is also clear that in the case of two equal angles, the downforce will have its maximum value among the various states in which the overall angle ($\alpha + \beta$) is constant and also the transverse forces will cancel each other.
Units of Froce:
$lb_{F}$ = Pound-Force
$kg_{F}$ = Kilogram-Force = $0.45359 \times lb_{F}$
$N$ = Newton = $9.80665 \times kg_{F}$
Strings Tension in $lb_{F}(kg_{F})$: (According to: Violin Strings Review)
| E String | A String | D String | G String | Full Set | |
| Dominant | 17.2(7.8017) | 12.1(5.4884) | 9.1(4.1277) | 9.9(4.4905) | 48.3(21.9084) |
| Vision Solo | 17.8(8.0739) | 12.1(5.4884) | 9.9(4.4905) | 10.1(4.5813) | 49.9(22.6341) |
| Evah Prazzi Gold | 17.6(7.9832) | 12.3(5.5792) | 10.4(4.7173) | 10.1(4.5813) | 50.4(22.8609) |
| Pirastro | 17.2(7.8017) | 12.8(5.8060) | 10.6(4.8081) | 11(4.9895) | 51.6(23.4052) |
Strings Downforce Calculator
Tension can also be derived through this formula:
$f = \frac{1}{2L}\sqrt[]{\frac{T}{\mu}}$
$f$ = Fundamental Frequency
$L$ = Length of the Vibrating Part of the String
$T$ = String Tension
$\mu$ = Linear Density (Mass Per Unit Length)